Quick Labs: AP Physics/Energy

The AP Physics class did a bang-up job on some quick labs about energy on Friday - just a mere 3 days before winter break!  All three projects showed terrific use of revision of their prototype experiments, as well.

The premise of the quick lab is to design, carry out, analyze, and present an experiment during a single 90-minute class period.  Presentations should be a single whiteboard.

The experiments:
Alex, Alex, Brandon 

• They took video of basketball shots, from launch to floor, and used video analysis to determine how much energy the net (all were 'swishes,' of course) took from the ball as it went through. 
• Revisions: initially using the first two points of the video to determine the launch velocity gave unrealistically high results, so they changed that part of the analysis to use kinematics to analyze the change in horizontal and vertical position and time taken (all easily measured from the video) from launch to goal in order to find the launch velocity.
• The boards (yes, they cheated and used two!):

Kawala and Kati

• They slid a small whiteboard eraser down a ramp, after which it plunged to the floor.  To determine the coefficient of kinetic friction between the ramp and the block, they used the fall distance and how far away the eraser landed from the table, along with the ramp length and angle.  This required marrying kinematics, conservation of energy, and the work-energy theorem, and 2.5 gallons of algebra.
• Revisions: they used two different 'floors' to take data, having the eraser hit the actual floor in one trial and hit a crate sitting on the floor in another trial.  The advantage of this is that we can find errors by looking at the trend in the data as the height of the floor is varied.  This is a great check that nothing unexpected is going on, and it was a good thing, because the first value was reasonable, but there were a few little errors to be uncovered.  The coefficients came out negative when the height was changed, so it gave a prompt to find the issues, and now they're confident in these numbers!
• The boards (also two!):

  

Cam, Mike, and Toru:

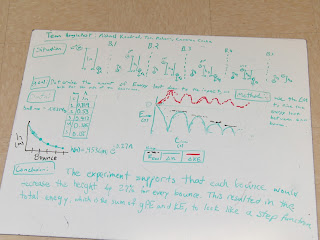
• These guys took some high-speed video as well, analyzing the heights of a bouncing ping-pong ball.  In addition to modeling the height of the ball as a function of bounce (and making a slick argument that the exponential function implies a constant-percentage loss of energy (rather than a constant amount of energy lost), they used Logger Pro to graph the gravitational PE, kinetic energy, and total energy as a function of time, showing the expected constant total energy during each flight (well, a slight decline due to air resistance), with "steps down" in total energy following each bounce.
• Revisions: after considering the parallax issue with measuring the height of the bouncing ball moving in front of a meter stick (especially with the camera mounted to a tripod), they instead dropped the ball next to the meter stick, eliminating the point-of-view issue.
• The board:

Why We Run Towards the Gunfire

We had some scheduling issues last week in physics with visitors, so I made an in-class assessment a take-home assessment.  One great thing about SBG is that there's really no point to cheating on such an assessment, so I think that I'm still getting a good picture of where they are.

On this question, they were (in general) in the weeds:

" Two radio towers 30 km apart transmit synchronized 240 kHz signals.  If a car equipped with a radio receiver tuned to the transmission frequency drives directly from one tower to the other, what will the receiver hear?  Explain; a diagram would help!  Radio signals are light waves that travel 300,000,000 meters per second.

What would the signal be like at a point along the drive that is 8750 meters from the first tower

How would the driving experience change if the radios’ frequencies were changed to 300 kHz?"

We've done some work with 2-source interference, from looking at the "overlapping ripples" diagrams to doing some predictions of frequency from two interfering sound waves given the locations of some points of constructive and destructive interference in the room.

This is the same concept, but a different-looking context, and that's where kids that haven't quite figured out the whole axiomatic reasoning thing have difficulty - yes it looks different, but we can still use the same principles to make predictions about what happens.

In particular, the big message for 2-source interference is that, even though both waves start in phase, they may not be in phase when they reach you, if you're different distances from the two sources.  The difference in travel distance determines the phases of the waves and whether they'll interfere constructively or destructively.  [This type of relationship is familiar: rates (relatives of differences) are famously difficult for students to intuitively grasp - see calculus!]

Back to the story, though:

I collected the assessments at the beginning of class and then posted this problem via projector.  I set the online stopwatch to five minutes and told them to come up with something coherent in their whiteboarding groups. There was a good discussion after that, and we made a lot of good connections.

...before that, though, there was an audible groan when I posted the problem.

Why?  It's a hard problem!  They've already wrestled with it for some period of time, felt anxiety that they were adrift about (da-dum!) an assessment problem, and here I was bringing it up again.

Here's the thing, though: you don't learn anything by running away from those difficult problems - you have to figure them out so that you can use that understanding in the future.  Denial is death in problem-solving.

Soldiers and police officers are incredible because they run towards gunfire, while the rest of us run away.  There's an anxiety-filled and dangerous situation, but they do the harder thing and confront it directly.

In physics (or learning in general), we have to run towards the gunfire too - you have to seek out and fix those misconceptions and misunderstandings.  It's anxiety-filled, too, but one student yesterday noticed a crucial difference between the two situations, when SBG is used: for us, the wounds aren't permanent.  

Not Proficient? No problem - wrestle with the problem, come back, and then you'll be whole again.  Using traditional grading that students are accustomed to, I totally understand why they get gunshy, even at the level of course selection.  Reminding them that this is a safer space for making mistakes has to be a constant occurrence because of that ingrained anxiety, but it's well worth the effort.

One of my favorite demos

I love the demonstration of two-source interference of sound waves - two speakers and a sine wave generator is all it takes to get one of those moments that kids remember for a long while after the course is over (now, if we can just get them to remember why it happens!).

The echoes in my lab aren't too bad, and we can actually map an interference pattern reasonably well, in large areas.  At some point, I'd like to try this in a big space and have them mark nodes (quiet points) and antinodes (loud points) with cones or something, and see if we can recreate the classic illustration.

In the meantime, we mapped out a few points that were relatively easily found:

At this point, we already knew all about order lines and how these loud and soft points came to be; we had analyzed a diagram of the interfering waves, determined where constructive and destructive interference were happening, and noticed the pattern in the 'lines' of nodes and antinodes (and even brought those shapes back to the base definition of a hyperbola!).

This was their first chance to apply that to a live problem: what's the frequency of that annoying hum that I'm playing through the speakers, anyway?  Are these measurements all that we need to determine that?  After all, there's nothing about time here at all, so determining the frequency seems daunting at first.

"What order line is that first soft point on?" is the real catalyzing question that I ask the groups a few minutes into their whiteboarding, if they haven't figured out how to apply the two-source model yet.

From there, there are a few steps of reasoning, some triangle manipulation, and some unit conversions needed.  The triangles and unit conversions shouldn't be an issue at this point, though they still are for some that haven't reached an unconscious competence level.  These assumed skills can really derail you. Especially if a problem is already difficult enough to tax your faculties to the max to begin with, adding a protracted wrestle with a unit conversion or diagramming effectively is likely to push your brain into a useless fried state (like okra).

Once we've connected that the order number is important, that connects to something about the distances from the speakers to the point, so we need to find those distances, using the Pythagorean theorem:

OK, great, but what does the order number tell us? This is a place where the sound is quiet because there's destructive interference, which happens because the waves travel different distances to get here.  The waves began synchronized, but since they've traveled different distances, they're at different points in their oscillation between high and low pressure (they're out of phase).  At this place, the wave from the left speaker has traveled half of a wavelength further than the wave from the right speaker (that's the .5 in the order number!).  Now we know two different ways to write the difference in travel distances:

 

Now that we know the wavelength, we're all set:

That's quiet a chain of thought there - let's trace the inferences that you have to make to solve this problem:

That's quite a set of inferences and observations, each necessary to solve the problem.  This number of inferences and observations is necessary for many problems that students try to solve, but we don't often think about the chain of logic so literally.  A great deal goes on behind the scenes... or doesn't.  Note too that there's knowledge from previous courses, previous terms and units of this course, and the current situation, but that it all has to fit together; this doesn't happen if students view understanding as disposable.  That sort of mindset is like tying your leg to a tree and trying to go for a run - no matter what direction you go, you can only go so far.

I think that, if students can get comfortable spelling out their reasoning like this, then they can get better at making a linear argument, which is what all problem-solving is - each step must be supported by knowledge or information and must lead to the next step.  Scatter-shot thinking is rampant, and it does not help problem-solving.  Perhaps conscious effort on constructing a "chain of reasoning" can help a student be able to do this type of thinking unconsciously, too!